Objective: Given a set of positive integers, and a value sum S, find out if there exists a subset in an array whose sum is equal to the given sum S.
Example:
int[] A = { 3, 2, 7, 1}, S = 6
Output: True, subset is (3, 2, 1}
We will first discuss the recursive approach and then we will improve it using Dynamic Programming.
Recursive Approach:
Every element in the array has two options, either we will include that element in the subset or we don’t include it.
- So if we take example as int[] A = { 3, 2, 7, 1}, S = 6
- If we consider another int array with the same size as A.
- If we include the element in the subset we will put 1 in that particular index else put 0.
- So we need to make every possible subset and check if any of the subsets makes the sum as S.
- If we think carefully this problem is quite similar to ” Generate All Strings of n bits”
- See the code for a better explanation.
Code:
Time Complexity: O(2n).Approach: Dynamic Programming (Bottom-Up)
Base Cases:
- If no elements in the set then we can’t make any subset except for 0.
- If the sum needed is 0 then by returning the empty subset we can make the subset with sum 0.
Given – Set = arrA[], Size = n, sum = S
- Now for every element in he set we have 2 options, either we include it or exclude it.
- for any ith element-
- If include it => S = S-arrA[i], n=n-1
- If exclude it => S, n=n-1.
Recursive Equation:
Base Cases:
SubsetSum(arrA, n, S)= false, if sum > 0 and n == 0 SubsetSum(arrA, n, S)= true, if sum == 0 (return empty set)
Rest Cases
SubsetSum(arrA, n, S) = SubsetSum(arrA, n-1, S)|| SubsetSum(arrA, n-1, S-arrA[n-1])
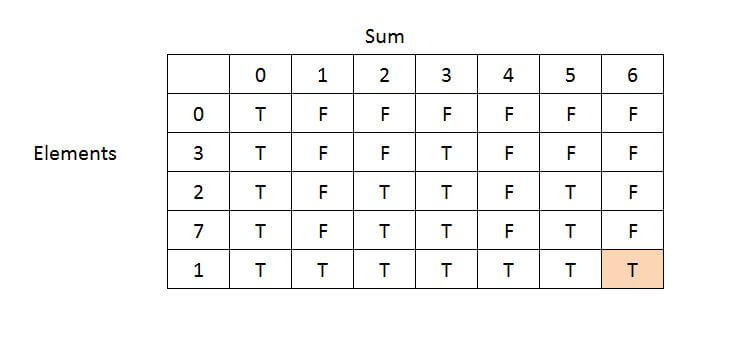
How to track the elements.
- Start from the bottom-right corner and backtrack and check from the True is coming.
- If the value in the cell above is false that means the current cell has become true after including the current element. So include the current element and check for the sum = sum – current element.
Code:
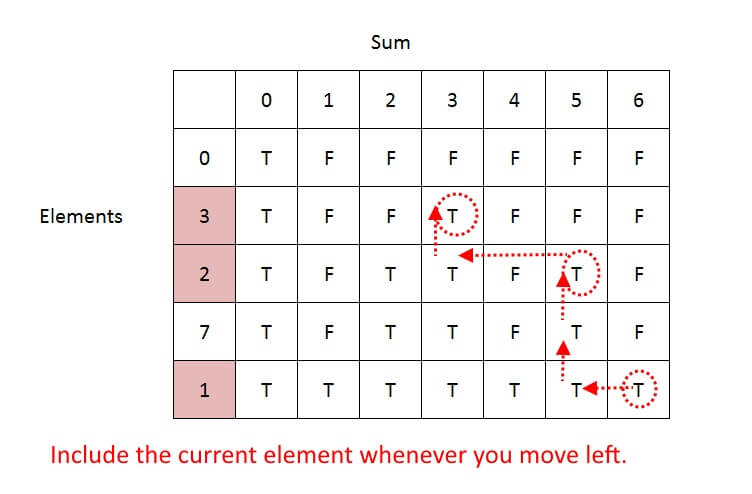
Output:
Output: From DP: true
my solution, prints all subsets with the required sum
http://www.edufyme.com/code?id=45c48cce2e2d7fbdea1afc51c7c6ad26
Doesn’t that algorithm have a terrible worst case scenario?
The problem statements are different. Print subset with required sum vs print *all* subsets with required sum. If you have a solution in mind which modifies the solution given on the page to print all subsets with lower time/space complexity; I would love to discuss.
Well, the problem was, check if there exists a subset. And the space complexity of the one given here could be reduced further by just keeping it in into one long long array with the size of the sum you want to add up to (O(S)). Gotta save it as numbers then, and not boolean.
In the recursive solution
solution[index] = 0;// do not select the element
currSum -= A[index]; //<<why this minus?.
Thank you so much for this solution.
I’m trying to print this page, but the sidebar on the right is blocking too much of the page, including half of the code blocks. Can you please fix this problem? Maybe you can provide a way to print where the right sidebar is removed? Thank you.
Hi, how do I trace it from the table when there is more than 1 subsets? For example, sum=5, array=[1,2,3,4,5]?
/*Recursive method*/
#include
using namespace std;
void subsetSum(int weights[],int target,int sum,vector v,int i,int n){
if(sum==target){
for(int i=0;i<v.size();i++){
cout<<v[i]<<" ";
}
cout<=n || sum>target){
return;
}
//include
v.push_back(weights[i]);
subsetSum(weights,target,sum+weights[i],v,i+1,n);
//exclude
v.pop_back();
subsetSum(weights,target,sum,v,i+1,n);
return;
}
int main(){
int weights[] ={ 7,8,9,11,20};
int target =20;
vector v;
subsetSum(weights,target,0,v,0,5);
return 0;
}