|
Be the first user to complete this post
|
Add to List |
286. Introduction to Minimum Spanning Tree (MST)
What is a Spanning Tree?
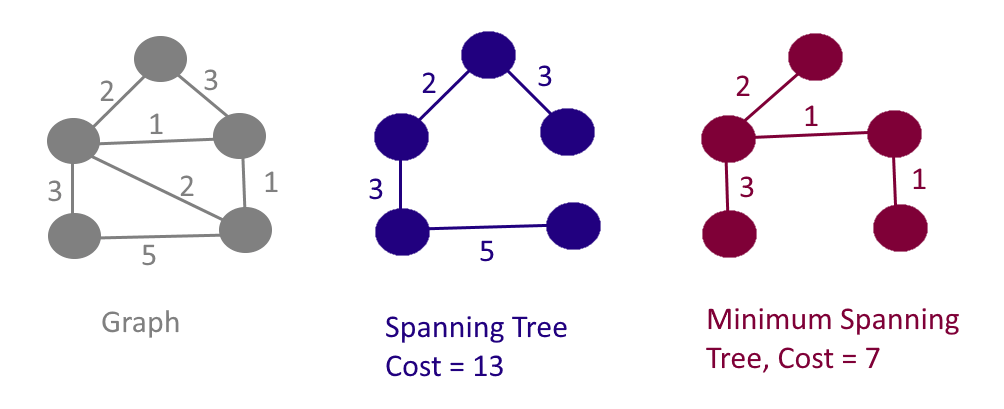
In an undirected and connected graph G=(V,E), a spanning tree is a subgraph that is a tree which includes all of the vertices of G, with minimum possible number of edges. A graph may have several spanning trees. The cost of the spanning tree is the sum of the weights of all the edges in the tree
What is a Minimum Spanning Tree?
A minimum spanning tree (MST) or minimum weight spanning tree is a subset of the edges of a connected, edge-weighted (un)directed graph that connects all the vertices together, without any cycles and with the minimum possible total edge weight. That is, it is a spanning tree whose sum of edge weights is as small as possible. Number of edges in MST: V-1 (V – no of vertices in Graph).
Example:
Algorithms for finding the Minimum Spanning Tree:
Applications:
Minimum spanning trees have direct applications in the design of networks, including computer networks, telecommunications networks, transportation networks, water supply networks, and electrical grids. Also used in
- Approximating travelling salesman problem
- Approximating multi-terminal minimum cut problem
- Approximating minimum-cost weighted perfect Cluster Analysis
- Handwriting recognition
- Image segmentation
- Circuit design
Reference - wiki

